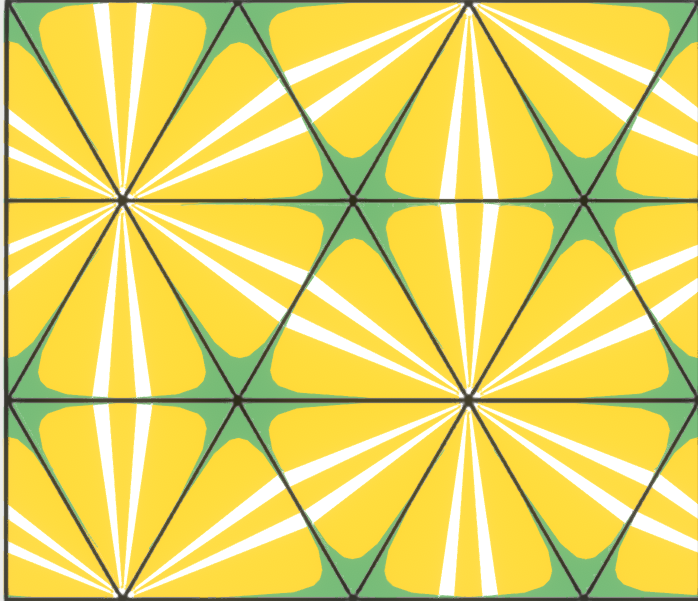
Catalog of tessellations
A tiling or tessellation on a plane is a motif that repeats itself in two dimensions. Such motifs appear frequently in architecture and art, particularly on textiles, tiles, and wallpaper. In mathematical terminology, each tessellation corresponds to a plane symmetry group. Below, we present the 17 possible tessellations, each labelled with its crystallographic name, and with the orbifold notation in parentheses. For each tessellation, we illustrate one way—but not the only one—of reproducing it [1].
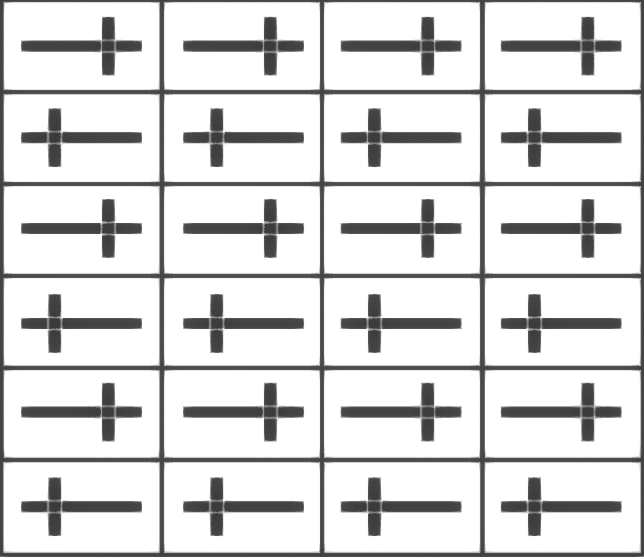
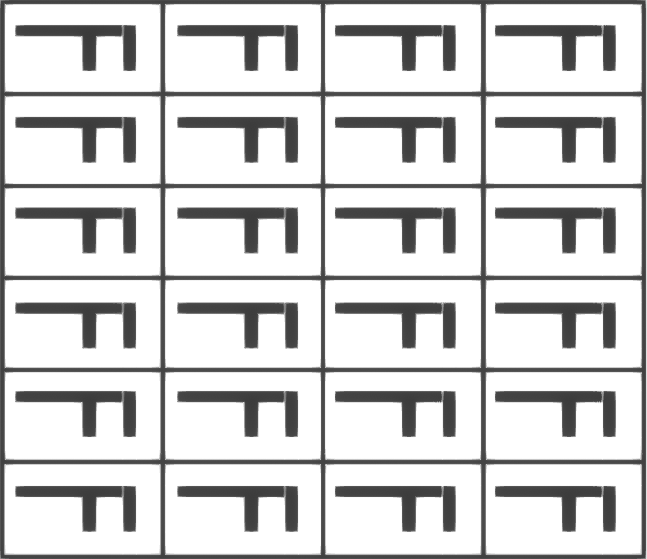
p1(O)
Shape to select:
square, rectangle or rhombus.
Internal configuration of the tile:
free, without internal symmetries.
Tessellation:
the tile is repeated identically through translations in both directions.

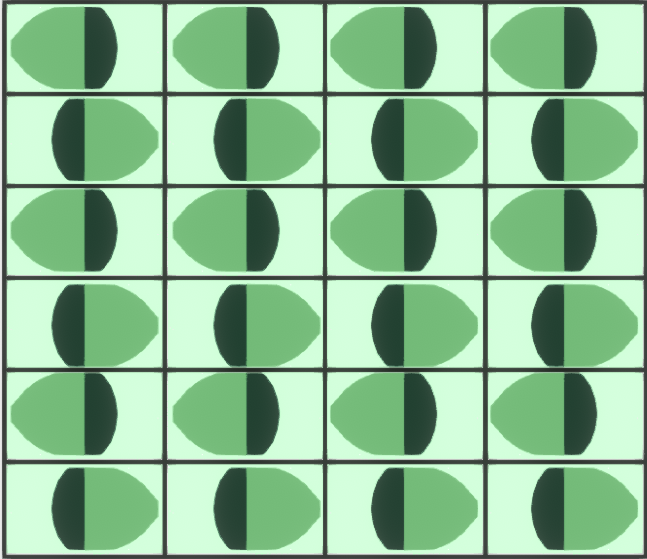
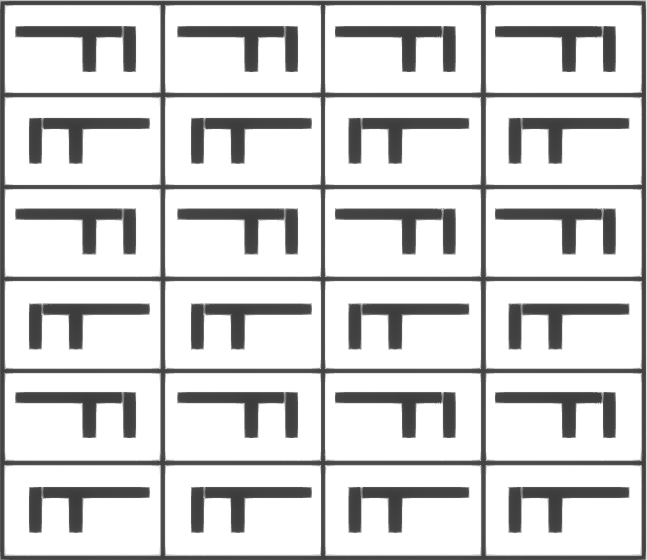
pg
Shape to select:
square or rectangle.
Internal configuration of the tile:
free, without internal symmetries.
Tessellation:
the tile is repeated identically through translations in one direction, whereas in the perpendicular direction, the tile is reflected with respect to the vector of translation.

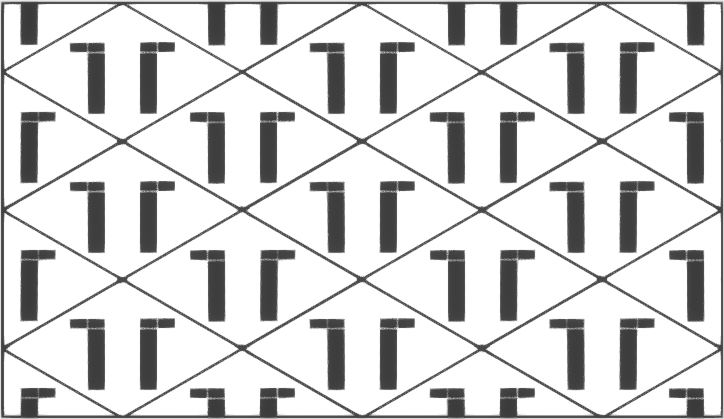
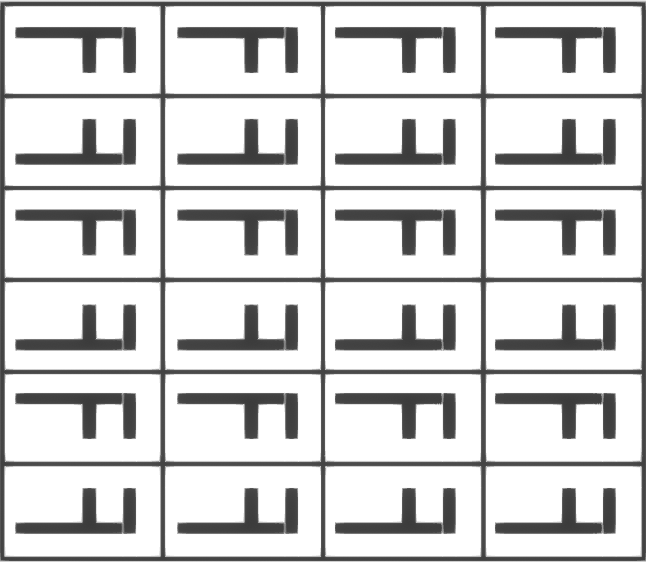
pm
Shape to select:
square or rectangle.
Internal configuration of the tile:
free, without internal symmetries.
Tessellation:
the tile is repeated identically through translations in one direction, whereas in the perpendicular direction, the tile is reflected.

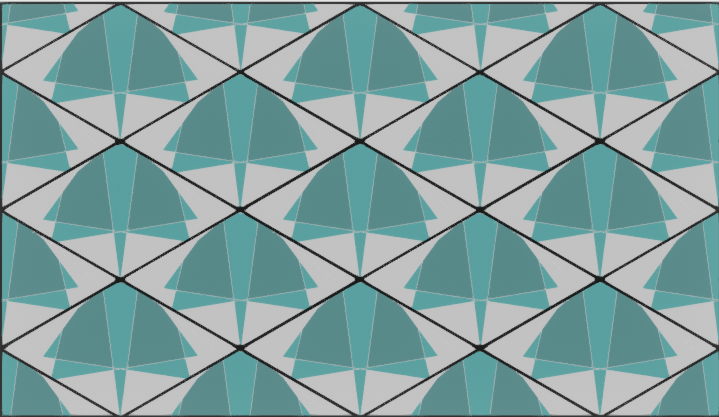
p2
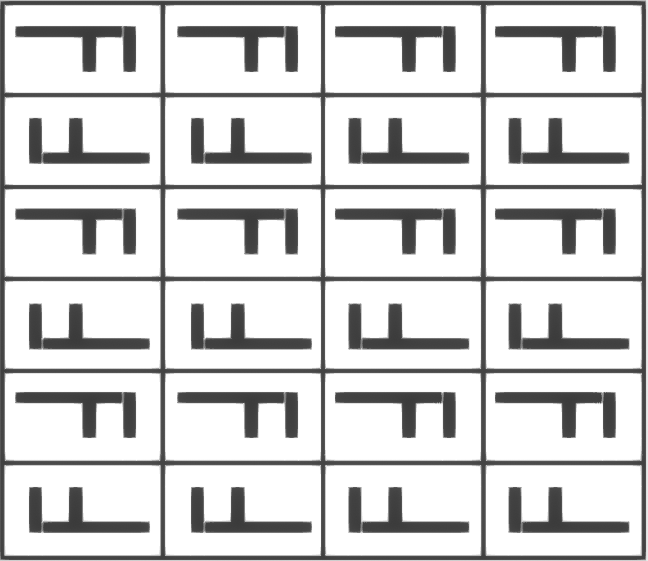
Shape to select:
square, rectangle or rhombus.
Internal configuration of the tile:
free, without internal symmetries.
Tessellation:
the tile is repeated identically through translations in one direction, whereas in the other direction, tiles are obtained through a point reflection.

pmm
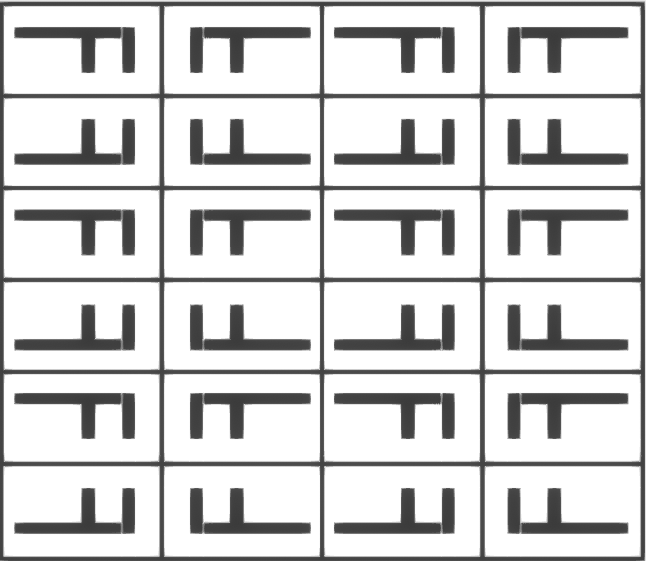
Shape to select:
square or rectangle.
Internal configuration of the tile:
free, without internal symmetries.
Tessellation:
the tile is reflected both directions.
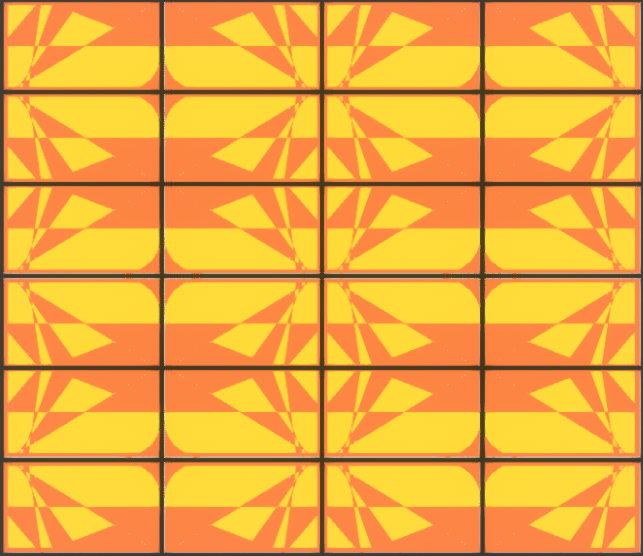
pmg
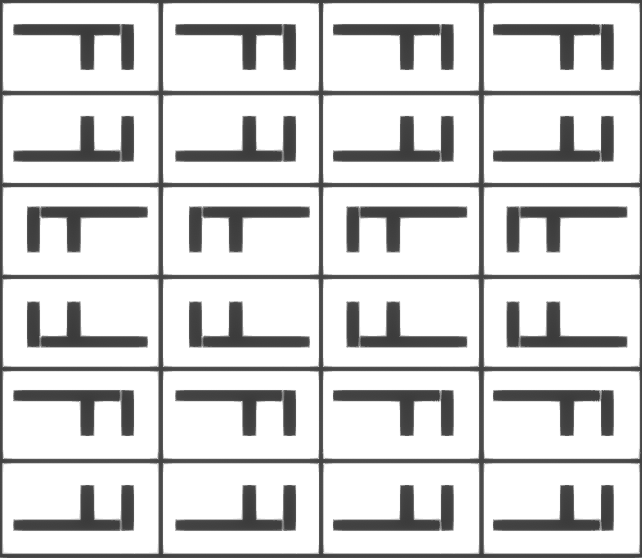
Shape to select:
square or rectangle.
Internal configuration of the tile:
symmetry with respect to a midpoint conjunction.
Tessellation:
the tile is repeated identically through translations in one direction, whereas in the perpendicular direction, you alternate between the tile being reflected and a point reflection being applied.